3. The Conditions for Triangle Similarity
Sign In
An error ocurred, try again later!
Chapter 2
3.
The Conditions for Triangle Similarity
Understanding the conditions for triangle similarity is pivotal in geometry. The lesson delves into the Angle-Angle Similarity Theorem, which states that if two angles of one triangle are congruent to two angles of another triangle, then the triangles are similar. Additionally, the Side-Side-Side Similarity Theorem is explored, emphasizing that if the corresponding sides of two triangles are proportional, the triangles are deemed similar. These conditions provide a foundation for determining the similarity of triangles, aiding in various geometric applications and problem-solving scenarios.
Show less Show more expand_more 
Lesson Settings & Tools
| | 11 Theory slides |
| | 11 Exercises - Grade E - A |
| | Each lesson is meant to take 1-2 classroom sessions |
The Conditions for Triangle Similarity
Slide of 11
In this lesson some conditions will be developed that guarantee the similarity of triangles.
Catch-Up and Review
Here are a few recommended readings before getting started with this lesson.
- The concept of similarity.
- Conditions for similarity of polygons.
- The concept of congruence.
- Conditions that guarantee the congruence of triangles.
Challenge
Share
Report error
Using Similarity of Triangles to Solve Problems
Find the ratio of the length of a diagonal and a side of a regular pentagon.
{"type":"text","form":{"type":"interval","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":true,"useShortLog":false,"variables":[],"constants":[]},"rendered":false,"decimal":true},"text":" "},"formTextBefore":"AC\/CD=","formTextAfter":null,"answer":{"text1":"1.618","ineq1":"le","text2":"1.62","ineq2":"le"}}
Explore
Share
Report error
Investigating Triangles With Two Pairs of Congruent Angles
Discussion
Share
Report error
Angle-Angle Similarity Theorem
Two polygons are similar if corresponding angles are congruent and corresponding sides are proportional. For triangles, the congruence of two angles already implies similarity.
If two angles of a triangle are congruent to two angles of another triangle, then the triangles are similar.
If ∠ A ≅ ∠ D and ∠ B ≅ ∠ E, then △ ABC ~ △ DEF.
Proof
Angle-Angle Similarity TheoremConsider two triangles △ ABC and △ DEF, whose two corresponding angles are congruent.
These triangles can be proven to be similar by identifying a similarity transformation that maps one triangle onto the other. First, △ DEF can be dilated with the scale factor k= ABDE about D, forming the new triangle △ DE'F'.
Since a dilation is a similarity transformation, it can be concluded that △ DE'F' and △ DEF are similar triangles. Next, it has to be proven that a rigid motion that maps △ DE'F' onto △ ABC exists. The corresponding angles of similar figures are congruent, so ∠ E' and ∠ E are congruent angles. ∠ E'≅ ∠ E Additionally, since ∠ E is congruent to ∠ B, by the Transitive Property of Congruence, ∠ E' is congruent to ∠ B. ∠ E'≅ ∠ B The ratios of the corresponding side lengths of similar polygons are the same and equal to the scale factor. DE'/DE=k In this case, the scale factor k is ABDE. Applying the Transitive Property of Equality, an equation can be formed and simplified. DE'/DE=AB/DE ⇕ DE'=AB It has been obtained that the two angles and the included side of △ DE'F' are congruent to the corresponding two angles and the included side of △ ABC.
Therefore, by the Angle-Side-Angle (ASA) Congruence Theorem, the two triangles are congruent. △ ABC≅△ DE'F' Since congruent figures can be transformed into each other using rigid motions, and △ ABC and △ DE'F' are congruent triangles, there is a rigid motion placing △ DE'F' onto △ ABC.
Therefore, it can be concluded that △ ABC and △ DEF are similar triangles.
△ ABC~ △ DEF
The proof is now complete.
Example
Share
Report error
Solving Problems Using Angle-Angle-Similarity Theorem
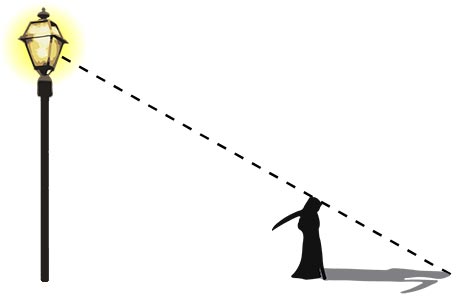
The Grim Reaper, who is 5 feet tall, stands 16 feet away from a street lamp at night. The Grim Reaper's shadow cast by the streetlamp light is 8 feet long. How tall is the street lamp?
{"type":"text","form":{"type":"math","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":true,"useShortLog":false,"variables":[],"constants":[]},"rendered":false},"text":" "},"formTextBefore":null,"formTextAfter":"feet","answer":{"text":["15"]}}
Hint
Both the lamp post and the Grim Reaper stand vertically on horizontal ground.
Solution
A sketch of the situation is helpful for finding the solution. Under the assumption that the lamp post and the Grim Reaper make right angles in relation to the ground, two right triangles can be drawn. The unknown height of the lamp post is labeled as x.
As these triangles both have a right angle and share the angle on the right-hand side, they are similar by the Angle-Angle (AA) Similarity Theorem. Notice that the base of the larger triangle measures to be 24 feet.
Since the triangles are similar, the ratios between corresponding side lengths are the same. \begin{gathered} \dfrac x 5 = \dfrac{24}8 \end{gathered} The solution of this equation defines the value of x — the height of the street lamp.
\dfrac x 5 = \dfrac{24}8
x = 15
The street lamp at 15 feet high towers over The Grimp Reaper.
Pop Quiz
Share
Report error
Practice Solving Problems Using Similar Triangles
For the given diagram, find the missing length.
Discussion
Share
Report error
Side-Side-Side Similarity Theorem
A second theorem allows for determining triangle similarity when only the lengths of corresponding sides are known.
If corresponding sides of two triangles are proportional, then the triangles are similar.
If AB/DE=BC/EF=CA/FD, then △ ABC ~ △ DEF.
Proof
Consider two triangles △ ABC and △ DEF, whose corresponding sides are proportional.
These triangles can be proven to be similar by identifying a similarity transformation that maps one triangle onto the other. First, △ DEF can be dilated with the scale factor k= ABDE about D, forming the new triangle △ DE'F'.
Because dilation is a similarity transformation, it can be concluded that △ DE'F' and △ DEF are similar triangles. Now, it has to be proven that a rigid motion that maps △ DE'F' onto △ ABC exists. The ratios of the corresponding side lengths of similar polygons are the same and equal to the scale factor. DE'/DE= DF'/DF= E'F'/EF=k In this case, the scale factor k is ABDE. Since all of the sides of △ ABC and △ DEF are proportional, the scale factor can be expressed by any of the following ratios. k= AB/DE= BC/EF= CA/DF Applying the Transitive Property of Equality, three equations can be formed and simplified. DE'/DE&=AB/DE [0.3cm] DF'/DF&=CA/DF [0.3cm] E'F'/EF&=BC/EF ⇒ DE' &= AB DF' &= CA E'F' &= BC These relations imply that the three sides of △ DE'F' are congruent to the three sides of △ ABC. Therefore, by the Side-Side-Side (SSS) Congruence Theorem, the two triangles are congruent. △ ABC≅△ DE'F' Since congruent figures can be transformed into each other using rigid motions, and △ ABC and △ DE'F' are congruent triangles, there is a rigid motion placing △ DE'F' onto △ ABC.
The combination of this rigid motion and the dilation performed earlier forms a similarity transformation that maps △ DEF onto △ ABC.
Therefore, it can be concluded that △ ABC and △ DEF are similar triangles.
△ ABC~ △ DEF
The proof is now complete.
Example
Share
Report error
Solving Problems Using Similar Triangles
There are four congruent angles in the figure. Try to identify them.
Answer
∠ DBA ≅ ∠ BCE ≅ ∠ BEC ≅ ∠ DBE
Hint
Look for similar triangles and an isosceles triangle.
Solution
Step 1
First, notice that segments BE and BC are equal in length.
These are two sides of △ BCE, so by the Isosceles Triangle Theorem, the opposite angles are congruent. ∠ BCE≅∠ BEC
Step 2
Two of the triangles, △ ABD and △ ACE look similar.
Because the lengths of the sides are given, the ratio of corresponding sides can be calculated.
| Ratio | Expression | Simplification |
|---|---|---|
| AC/AB | 459+1275/459=1734/459 | 34/9 |
| AE/AD | 405+1125/405=1530/405 | 34/9 |
| EC/DB | 1360/360 | 34/9 |
The last column of the table shows that the corresponding sides of △ ACE and △ ABD are proportional. AC/AB=AE/AD=EC/DB According to the Side-Side-Side (SSS) Similarity Theorem, the two triangles are similar. △ ACE~△ ABD Corresponding angles of similar triangles are congruent.
One pair of these angles is marked on the figure. ∠ BCE≅∠ DBA
Step 3
In addition to the proportions in Step 2 showing that △ ACE and △ ABD are similar, they also show the two triangles are dilations of each other from the common vertex A. Since dilations map a segment to a parallel segment, segments DB and EC are parallel.
Furthermore, since EB is a transversal to two parallel lines, the Alternate Interior Angles Theorem guarantees that the angles at E and B are congruent. ∠ BEC≅∠ DBE These angles are marked on the figure.
Answering the Question
The previous three steps showed three pairs of congruent angles. The transitive property of congruence shows that all four angles mentioned in these pairs are congruent to each other. ∠ DBA ≅ ∠ BCE ≅ ∠ BEC ≅ ∠ DBE The congruent angles are marked on the figure.
Discussion
Share
Report error
Side-Angle-Side Similarity Theorem
Two theorems have been covered, now a third theorem that can be used to prove triangle similarity will be investigated. This third theorem allows for determining triangle similarity when the lengths of two corresponding sides and the measure of the included angles are known.
If two sides of a triangle are proportional to two sides of another triangle and the included angles are congruent, then the triangles are similar.
If AB/DE=AC/DF and ∠ A ≅ ∠ D, then △ ABC ~ △ DEF.
Proof
Consider two triangles △ ABC and △ DEF, whose two pairs of corresponding sides are proportional and the included angles are congruent.
These triangles can be proven to be similar by identifying a similarity transformation that maps one triangle onto the other. First, △ DEF can be dilated with the scale factor k= ABDE about D, forming the new triangle △ DE'F'.
Because dilation is a similarity transformation, it can be concluded that △ DE'F' and △ DEF are similar triangles. Now, it has to be proven that a rigid motion that maps △ DE'F' onto △ ABC exists. The ratios of the corresponding side lengths of similar polygons are the same and equal to the scale factor. DE'/DE= DF'/DF=k In this case, the scale factor k is ABDE. Since AB and AC are proportional to DE and DF respectively, the scale factor can be expressed by any of the following ratios. k= AB/DE= AC/DF Applying the Transitive Property of Equality, three equations can be formed and simplified. l c r DE'/DE=AB/DE & ⇒ & DE'=AB [0.3cm] DF'/DF=AC/DF & ⇒ & DF'=AC These relations imply that the two sides of △ DE'F' are congruent to the corresponding two sides of △ ABC. Moreover, the included angles ∠ A and ∠ D are also congruent.
Therefore, by the Side-Angle-Side (SAS) Congruence Theorem, the two triangles are congruent. △ ABC≅△ DE'F' Since congruent figures can be transformed into each other using rigid motions, and △ ABC and △ DE'F' are congruent triangles, there is a rigid motion placing △ DE'F' onto △ ABC.
The combination of this rigid motion and the dilation performed earlier forms a similarity transformation that maps △ DEF onto △ ABC.
Therefore, it can be concluded that △ ABC and △ DEF are similar triangles.
△ ABC~ △ DEF
The proof is now complete.
Example
Share
Report error
Proving Similarity Between Triangles Given Sides
The diagram shows the distances between points on a figure.
Show that △ ABC and △ AED are similar triangles. Then find DE.
{"type":"text","form":{"type":"math","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":true,"useShortLog":false,"variables":[],"constants":[]},"rendered":false},"text":" "},"formTextBefore":"DE=","formTextAfter":"units","answer":{"text":["6"]}}
Hint
Solution
Triangles △ ABC and △ AED have a common angle at A.
The table below contains the ratios of two pairs of corresponding sides of the two triangles.
| Ratio | Expression | Simplified Form |
|---|---|---|
| AB/AE | 7/5+9=7/14 | 1/2 |
| AC/AD | 5/7+3=5/10 | 1/2 |
Closure
Share
Report error
Applying Triangle Similarity Theorems to Solve Problems
Through applying the theorems of similar triangles, the ratio of the lengths of a diagonal and the sides of a regular pentagon can be found.
{"type":"text","form":{"type":"interval","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":true,"useShortLog":false,"variables":[],"constants":[]},"rendered":false,"decimal":true},"text":" "},"formTextBefore":"AC\/CD=","formTextAfter":null,"answer":{"text1":"1.618","ineq1":"le","text2":"1.62","ineq2":"le"}}
Hint
Begin by determining the angle measures of the figure.
Solution
The Polygon Angle Sum Theorem identifies the sum of the interior angle measures of a pentagon. (5-2)180=540 In a regular pentagon, all five angles are equal measures. Therefore, one of the measures of the angles is a fifth of the sum of the five angle measures. m∠ A = 540/5=108 Furthermore, since the sides of a regular pentagon are congruent, △ ABE is an isosceles triangle.
m∠ ABE + m∠ AEB + m∠ A = 180
SubstituteII
m∠ AEB= m∠ ABE, m∠ A= 108
m∠ ABE + m∠ ABE + 108 = 180
▼
Solve for m∠ ABE
SubEqn
LHS-108=RHS-108
m∠ ABE + m∠ ABE = 72
SumToProdTwoTerms
a+a=2a
2m∠ ABE = 72
DivEqn
.LHS /2.=.RHS /2.
m∠ ABE = 36
m∠ BAC + m∠ CAD + m∠ DAE = m∠ A
SubstituteValues
Substitute values
36 + m∠ CAD + 36 = 108
SubEqn
LHS-(36+36)=RHS-(36+36)
m∠ CAD = 36
Next, focus on △ ACE. In this triangle, AC and EC are diagonals of the pentagon, and AE is a side.
In the diagram, a smaller triangle labeled △ AFE is also present. These two triangles share a common angle at A and congruent angles at C and E. ∠ CAE&≅∠ EAF ∠ ACE&≅∠ AEF According to the Angle-Angle (AA) Similarity Theorem, that means the two triangles are similar. Therefore, the corresponding sides are proportional. CA/AE=AE/AF Continuing forward, notice that triangles △ AFE and △ CEF are isosceles. Therefore, their legs have equal lengths. AE=EF=FC Using s for the length of the sides, AE=EF=FC=s, as indicated on the figure. Also, using d for the length of the diagonal, CE=d and FA=CA-FC=d-s.
x=1/x-1
▼
Solve for x
MultEqn
LHS * (x-1)=RHS* (x-1)
x(x-1)=1
MultPar
Multiply parentheses
x^2-x=1
SubEqn
LHS-1=RHS-1
x^2-x-1=0
UseQuadForm
Use the Quadratic Formula: a = 1, b= - 1, c= - 1
x=-( -1)±sqrt(( -1)^2-4( 1)( -1))/2( 1)
NegNeg
- (- a)=a
x=1±sqrt((-1)^2-4(1)(-1))/2(1)
CalcPowProd
Calculate power and product
x=1±sqrt(1-(-4))/2
SubNeg
a-(- b)=a+b
x=1±sqrt(1+4)/2
AddTerms
Add terms
x=1±sqrt(5)/2
Length of diagonal/Length of side=1+sqrt(5)/2≈ 1.618
Extra
Construction of a regular pentagonThe ratio of the diagonal to the side of a regular pentagon can be used to prove that the following construction creates a regular pentagon. This is a construction created by Yosifusa Hirano in the 19th century.
The Conditions for Triangle Similarity
Exercise 3.1
You have a white rectangular shaped sheet of paper with a gray backside. The paper is folded such that the lower right corner meets the upper horizontal side and the crease goes through the lower left corner.
{"type":"text","form":{"type":"math","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":true,"useShortLog":false,"variables":["x"],"constants":["PI"]},"rendered":false},"text":" "},"formTextBefore":null,"formTextAfter":"cm^2","answer":{"text":["56"]}}
security
report
code
security
report
code
Let's first draw a diagram showing how the paper will look like when folded. At the same time, we will introduce some labels to make it easier to refer to the diagram.
In order to calculate the area of △ DEF, we need to know two side lengths. That means we need the length of EF. From the diagram, we see that x makes the leg of a right triangle where the hypotenuse and second leg are known. Therefore, we can determine x by using the Pythagorean Theorem.
When we know the length of the side labeled x, we can determine the length of EB by subtracting this from 15. 15-9 = 6 Examining the diagram, we can identify two right triangles, △ AED and △ EBF. We need to prove that these triangles are similar. Let's label the acute angles of these triangles.
At point E we can see that the sum of u, v and the right angle makes a straight angle. Therefore, we can write the following equation. m∠ u +m∠ v+90^(∘)= 180^(∘) ⇓ m∠ u = 90^(∘)- m∠ v Next, we will write an equation for the sum of the angles in △ AED. Then we can use the relationship just found to show that ∠ v and ∠ w are congruent.
As we have shown, ∠ w and ∠ v are congruent. Furthermore, since △ ADE and △ BFE have two pairs of congruent angles, we can claim similarity by the Angle-Angle Similarity Theorem.
Using this similarity, we can write an equation preparing to solve for EF.. EF/15=6/12 Let's go for it!
When we know the length of EF, we can calculate the gray triangle's area.
The visible gray area is about 56 square centimeters.
security
report
code
The city council is planning to build a school on a plot of land. Below we see a diagram of the school as seen from above. The school's two shorter buildings each have an entrance facing the courtyard. These are labeled E_1 and E_2.
{"type":"text","form":{"type":"math","options":{"comparison":"1","nofractofloat":false,"keypad":{"simple":false,"useShortLog":false,"variables":["x"],"constants":["PI"]},"rendered":false},"text":" "},"formTextBefore":null,"formTextAfter":"feet","answer":{"text":["250\\sqrt{2}"]}}
security
report
code
security
report
code
Let's start by drawing an outline of the school and adding some lengths to the buildings. We will also label the buildings as A, B, and C.
To determine the minimal distance, we remember that the shortest distance between two points is a straight line. Therefore, we need to place E_3 somewhere along the side of the longest building such that the distance from E_1 to E_3 to E_2 is as short as if it was a straight line. To find where we must place E_3, we can reflect E_2 across the longer side of B.
The distance from E'_2 to E_1 is minimized by a straight segment between them. Where this segment intersects the longer side of B, is where we should place the third entrance to minimize the distance. If we draw this segment we can identify two similar triangles by the Angle-Angle Similarity Theorem.
Using the similarity between the triangles, we can write an equation. x/250-x=100/150 Let's solve this equation for x.
The third door should be placed 100 feet to the left of where B and C meet on the courtyard.
Now we can determine the minimum distance by using the Pythagorean Theorem. 150^2+150^2=c^2 &⇔ c =150sqrt(2) 100^2+100^2=d^2 &⇔ d=100sqrt(2) Finally, we will add the distance of c and d to obtain the minimal distance between the three entrances. c+d=150sqrt(2)+100sqrt(2) ⇓ c+d=250sqrt(2)feet
security
report
code
The Conditions for Triangle Similarity
Recommended exercises
To get personalized recommended exercises, please login first.
Loading content
Edit Lesson
≤
>
■2
■▢
e▢
7
8
9
×
÷■1
=
=
√▢
▢√
∫▢▢
4
5
6
+
−
≤
<
log
ln
log▢
1
2
3
()
∣
sin
cos
tan
0
.
π
x
y
Loading content