MathReads
Get Premium
Dashboard

Dashboard Sign out
A1
Algebra 1 View details
8. Levels of Accuracy
Continue to next lesson
Lesson
Exercises
Tests
arrow_back
eCourses /
Algebra 1 /
Chapter 1
8. 

Levels of Accuracy

This lesson focuses on the importance of accuracy in mathematics, specifically through the lens of rounding numbers, significant figures, absolute error, and percent error. Understanding these concepts is crucial for both academic and real-world applications. For example, in scientific research, even a small error can have significant implications. Similarly, in everyday life, rounding errors can affect everything from your bank balance to engineering calculations. The lesson provides step-by-step methods to round numbers to specific decimal places or significant figures and explains how to calculate and interpret both absolute and percent errors. This knowledge is invaluable for students, professionals, and anyone who deals with numbers regularly.
Show more expand_more
Lesson Settings & Tools
16 Theory slides
10 Exercises - Grade E - A
Each lesson is meant to take 1-2 classroom sessions
Image Credits expand_more
Levels of Accuracy
Slide of 16
Each digit is essential when measuring or reporting quantities such as temperatures, distances and speeds. Simply discarding certain digits can lead to errors and mistakes in results. Therefore, it is important to learn how to round numbers correctly to ensure the results are accurate.

Catch-Up and Review

Here are a few recommended readings before getting started with this lesson.

Here are a few practice exercises before getting started with this lesson.

a Pair each number with its corresponding number of decimal places.
b Pair each number with its corresponding number of significant figures.
Explore

Conflicts When Rounding Angle Measures

When rounding is not accurate enough, it can produce conflicting results. For example, the angle measures in the following triangle have been rounded to the nearest integer. As a result, the sum of the angle measures is not always equal to Move the vertices to see how the sum changes.
Triangle with angle measures rounded to the nearest integer
In this case, each number was rounded to avoid using decimals. However, keep in mind that even with the use of decimals, incorrect results may still occur.
Discussion

Meaningful Decimal Places

There are different scenarios where not all digits of a number are meaningful. In these cases, the number of decimal places required is usually given. However, there are cases where the number of decimal places needed can be deduced from the problem's context.

Example situations where having decimals makes no sense

In any case, it is important to know how to round a number to a specific number of decimal places.

Method

Rounding to Decimal Places

Rounding a decimal number to decimal places means to rewrite the number in a simpler and shorter form with exactly decimals. The final result will be an approximation to the given number.

The number rounded to two decimal places is

The following steps should be followed to round a number to decimal places.

1
Look For the th Digit to the Right of the Decimal Point
expand_more

Consider the number In each column, the corresponding decimal place is highlighted.

Round to One Decimal Place Round to Two Decimal Places Round to Three Decimal Places
2
Draw a Vertical Bar to the Right of This Digit
expand_more
Round to One Decimal Place Round to Two Decimal Places Round to Three Decimal Places
3
Look at the Digit to the Right of the Bar
expand_more
  • If it is less than keep the digit the same.
  • If it is equal to or more, increase the digit by
    • If the digit becomes write instead and increase the preceding digit by Repeat this as many times as needed.
Round to One Decimal Place Round to Two Decimal Places Round to Three Decimal Places
4
Remove the Bar and Any Digits to Its Right
expand_more

The following table shows the results of rounding to one, two, and three decimal places.

Round to One Decimal Place Round to Two Decimal Places Round to Three Decimal Places
Example

Making a Painting's Size as Precise as Possible

Mrs. Mathematiks asked Izabella to paint the Hubble Telescope on the classroom wall. Izabella thinks that she should draw a grid before doing the actual painting. She is told that squares with a side length of centimeters will work well, but she only has this ruler.

Ruler
According to this ruler, determine what side length Izabella could use to draw the square accurately. In this situation, it is not possible to measure exactly centimeters.

Hint

Since the ruler only shows the measurements down to the tenth of a centimeter, round the original length to the tenth value, one decimal place.

Solution

The exact side length of the square Izabella wants to draw is given to the thousandth of a centimeter. Therefore, it has a precision of three decimal places.

The number with all the place values labeled

Because the ruler measures to the tenth of a centimeter, Izabella cannot draw the square using the exact dimensions. To draw the square precisely, she must round the original length to a precise length that the ruler can measure.

The exact length must be rounded to decimal place.

Next, apply the steps to round a decimal number.

  1. Look for the digit to the right of the decimal point.
  2. Draw a vertical bar to the right of this digit.
  3. Look at the digit to the right of the bar.
  4. Since is greater than the digit, must be increased by It then becomes
  5. Remove the bar and all digits to its right.

In conclusion, Izabella should use a length of centimeters to precisely draw the square's sides.

Square of side length 3.7cm

Extra

 Izabella's Painting of the Hubble Telescope Using a Grid

Izabella used a pencil to draw an outline of the previously measured square to make a grid layout of many squares. Doing this helped her paint the telescope accurately. The image shows how the grid helped her painting process.

Telescope mural showing a grid used as a template
External credits: NASA Source modified by Jeremy
Pop Quiz

Rounding Different Numbers to Certain Decimal Places

Round the given number to the specified number of decimal places.

Random decimal numbers to be rounded to a specified number of decimal places.
Discussion

Learning About Significant Figures

When announcing lottery results, an approximation of the winnings is generally used instead of listing the actual amount of money won.

Example situation where a number has been rounded to 1 significant figure

In this case, the actual amount written in the title has been rounded to have only one significant figure. Rounding to significant figures is very similar to rounding to decimal places except for the final step.

Method

Rounding to Significant Figures

Rounding a number to significant figures means to rewrite the number so that it has exactly significant figures. The result is an approximation of the number.

The number rounded to three significant figures becomes

The following steps should be followed to round a number to significant figures.
1
Look For the Significant Figure
expand_more

Consider the number In each column, the corresponding significant figure is highlighted.

Round to Three Significant Figures Round to Five Significant Figures Round to Six Significant Figures
2
Draw a Vertical Bar to the Right of This Digit
expand_more
Round to Three Significant Figures Round to Five Significant Figures Round to Six Significant Figures
3
Look at the Digit to the Right of the Bar
expand_more
  • If it is less than keep the digit the same.
  • If it is or more, increase the digit by
    • If the digit becomes write instead and increase the previous digit by Repeat this as many times as needed.
Round to Three Significant Figures Round to Five Significant Figures Round to Six Significant Figures
4
Look at the Digits to the Right of the Significant Figure
expand_more
  • Remove the bar.
  • From the digit, any digit to the left of the decimal point must be replaced with zero.
  • From the digit, any digit to the right of the decimal point must be removed.

The following table shows the results of rounding to and significant figures.

Round to Three Significant Figures Round to Five Significant Figures Round to Six Significant Figures

Extra

 When The Rounded Number Has Fewer Digits Than the Original

It may happen that rounding a number results in an integer with fewer digits than the number of digits of the integer part of the original number. In this case, a bar is drawn above the last significant digit to indicate that there were more significant digits.

Rounding the Number to
Two Significant Figures Three Significant Figures Four Significant Figures
or or or

Alternatively, writing the number using scientific notation helps identify the number of significant figures.

Example

Participants in a Marathon

Mrs. Mathematikz has another student named Paulina, who loves running and public speaking. Mrs. Mathematikz talked a local sports radio network into letting Paulina be a guest host! Paulina is told that people signed up for a marathon. She is asked to round the number to two significant figures and to announce the rounded number to the listeners.
A reporter with a microphone and a group of people running behind
What number of participants will Paulina announce on the radio?

Hint

In the last rounding step, the numbers to the right of the second significant figure and the left of the decimal point must be replaced with zeros.

Solution

According to her boss, Paulina must round the number to significant figures. This can be done by following the steps below.

  1. Look for the significant figure.
  2. Draw a vertical bar to the right of this digit.
  3. Look at the digit to the right of the bar.
  4. Since is less than the second significant figure remains the same.
  5. Look at the digits to the right of the significant figure. From the second significant figure, any digit to the left of the decimal point must be replaced with zero.

Paulina will announce on the radio that about people have signed up for the marathon.

Discussion

Rounding Numbers Less Than or Numbers With Zeros in the Middle

Rounding to significant figures must be done carefully, particularly when the number is less than or has zeros in the middle. A step-by-step example of each case is illustrated below.
Rounding a number less than 1 or containing zeros in the middle to a specific number of significant figures
Note that the zeros are not significant in one case but they are significant in the other.
Pop Quiz

Rounding Different Numbers to Certain Significant Figures

Round the given number to the specified number of significant figures.

Random numbers to be rounded to a specified number of s.f.
Discussion

Performing Computations With Significant Figures

When carrying out computations with significant figures, scientists usually use two different methods for rounding off a final answer. One method is used for addition and subtraction, while the other is used for multiplication and division.

Addition or Subtraction Multiplication or Division
Round the result to the least number of decimal places in the numbers involved in the calculation. Round the result to the least number of significant figures in the numbers involved in the calculation.

The following table shows how to round the sum and the product of the numbers and Notice that the least number of decimal places is two and the least number of significant figures is four.

Computation Rounded Result
( decimal places)
( significant figures)
Discussion

Accuracy and Precision

The words accuracy and precision play an important role when doing measurements and rounding. Accuracy refers to the closeness of the measurements to the exact, known, or acceptable value. Precision is the closeness of the measurements to each other.

Three Targets with some darts showing the difference between precision and accuracy.

The word precision is also used to indicate the number of decimal places of a number. Moreover, it can be used to determine which of two numbers is given with more detail.

  • The number is given with a precision of three decimal places.
  • Between the numbers and the second is more precise because it has more decimal places.
Since rounding-off gives an approximation of the actual number, there is always a particular error called absolute error. Depending on the magnitude of this error, the final result could be acceptable or not.
Discussion

Absolute Error

The absolute error is the absolute value of the difference between a measured value and the exact value. The units of the absolute error correspond to the units of the measures. By definition, the absolute error is always greater than or equal to

Note that the absolute error gives only the distance between the measurements but does not say how significant the error is. For example, consider a professional basketball game. Suppose that the expected number of attendees was but the actual number of people in attendance is
Here, the absolute error is attendees, but it is unknown whether this error is big or small.
Example

Finding Absolute Errors

Eager to dish out another challenge, Mrs. Mathematikz gave Izabella, Paulina, and another friend Jordan the following problem.

find the value of the numeric expression 3.32 + 4.28*2.873. Using the scientist's methods of rounding to significant figures, round the result to three significant figures.
The three friends solved the problem separately and came up with the following results.
On Monday, Mrs. Mathematikz said that the value of the expression, rounded to three significant figures, is
a Pair each student with their corresponding absolute error.
b Who got the most accurate result?

Hint
a Find the absolute value of the difference between the result obtained by each student and the number given by the teacher.
b The most accurate result is the one with the least absolute error.

Solution
a The absolute error is the absolute value of the difference between the obtained result and the exact number.
Then, the absolute error made by Izabella is the absolute value of the difference between her result and the value given by the teacher.
▼
Substitute values and evaluate
SubstituteII

,

SubTerms

Subtract terms

AbsPos

The absolute error made by Izabella was Similarly, the absolute errors made by Paulina and Jordan can be calculated.
Absolute Error
Izabella
Paulina
Jordan
b From the table found in Part A, the least absolute error is the one made by Jordan.
As determined, Jordan obtained the most accurate result.
The absolute error does not necessarily indicate the importance of the error. For example, having an absolute error of one millimeter for a pizza's size is not as important as having an absolute error of one millimeter for the diameter of a contact lens prescription. In this case, the relative error is a better indicator.
Discussion

Relative Error and Percent Error

The relative error is the ratio of the absolute error of a measurement to the exact value. The relative error tells how good a measurement is relative to the size of the object being measured. In other words, the relative error indicates how significant the absolute error is.

Relative Error

The Relative Error Formula can be rewritten by substituting the Absolute Error Formula.

Relative Error

The percent error is the product between the relative error and It represents the relative error as a percentage.

Percent Error

There are scenarios where the absolute error seems to indicate that one result is more accurate than another. However, this can only be concluded by comparing the relative or percent errors.
Example

Absolute Error vs. Percent Error

Paulina did such a great job as a radio host that she is invited to announce another smaller race.

She notices something interesting about the runners at this smaller race — some are wearing headbands, and some are wearing sunglasses. She decides to take note of her observation to share it on the radio as a fun fact.

Headband Sunglasses
Number of Counted Runners With an Accessory
Actual Number of Runners With an Accessory
a What is the percent error made by Paulina concerning her count of headband runners? Round the number to one decimal place.
b For Paulina's count of headbands and sunglasses, which is the most accurate measure?

Hint
a Begin by finding the absolute error for headbands. Then, find the relative error. Finally, convert the relative error to percent form.
b Find the percent error made about sunglasses and compare it to the error made about headbands. The accessory with the least percent error is the most accurate measure.

Solution
a Start by finding the absolute error about runners wearing headbands.
▼
Substitute values and simplify
SubstituteII

,

SubTerms

Subtract terms

AbsNeg

To find the relative error, divide the absolute error by the corresponding exact value.
Finally, to compute the percent error, multiply the relative error by and round the result to one decimal place as requested.
As a result, the error for runners wearing a headband is about
b To determine which accessory count is the most accurate measure, the percent error for each one needs to be compared. Begin the comparison by finding the absolute error made by Maya.
▼
Substitute values and simplify
SubstituteII

,

SubTerms

Subtract terms

AbsNeg

Following the reasoning made in Part A, the absolute error will be divided by the corresponding exact value to find the relative error made by Maya.
Finally, multiply the relative error by to obtain the percent error and round the result to one decimal place.
Looking at the percent errors made about sunglasses and headbands, it can be seen that the percent error made about sunglasses is smaller.
Despite having the larger absolute error, the count concerning sunglasses was more accurate than the count concerning headbands. This demonstrates the importance of measuring the percent error.
Considering the previous examples, errors by only a few decimal places may not appear to be have such large consequences. In practice, however, these errors can lead to notoriously big complications.
Closure

Errors in Real Life

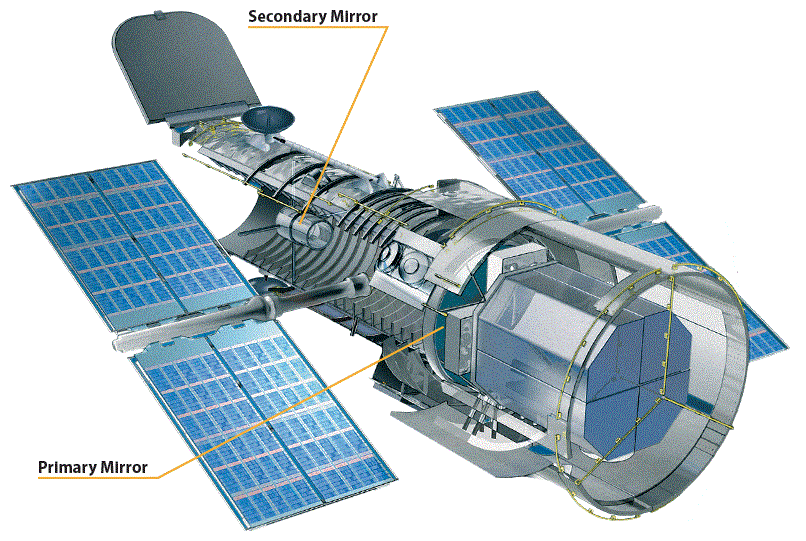
The Hubble Space Telescope is a telescope the size of a school bus that travels around Earth. Scientists and astronomers have learned a lot about space from Hubble's pictures of stars, planets, and galaxies.

Hubble-Telescope.gif

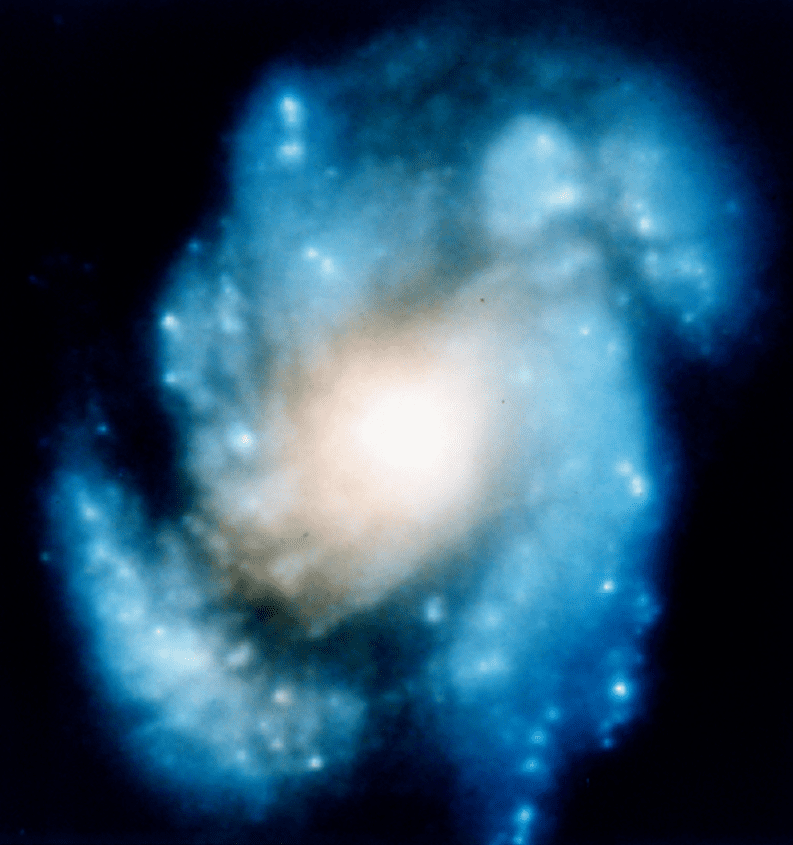
After its launch in NASA found an error in the primary mirror that made the first few images appear blurrier than expected. It turned out that the curvature of the mirror was deflected by less than one-millionth of a meter — just one-fiftieth the width of a human hair! The following photo, taken in November shows the core of the galaxy

Hubble-Picture-Core-M100-Blurry.png

NASA's first solution was to build replacement instruments — similar to a pair of prescription lenses — to fix the flaw. These repairs were done in December of The picture below, taken after the repairs, is the same galaxy shown in the first photo. What a difference!

Hubble-Picture-Core-M100-Corrected.png

This case shows that even though the error was a mere one-fiftieth of the width of a human hair, the final result was significantly impacted by the error.



Level 1
Level 2
Level 3

How many significant figures does the number have?

All digits that are non-zero are significant figures. Examining the number, we notice that it does not contain any zeros. Therefore, all of the digits are significant figures. 46 893 [-1.2em] Significant Not significant We have five significant figures.

Here, we have at least two significant figures, as two digits are not zero. Note that zeros can also be significant figures if they are between two non-zero digits or at the end of a decimal number. 0. 00 75 [-1.2em] Significant Not significant In this case, the zeros are at the beginning of the number. Therefore, we have only two significant figures.

Here, we have three digits that are non-zero and are therefore significant figures. However, we also have a zero that is between two non-zero digits. This is also a significant figure. 5. 0 54 [-1.2em] Significant Not significant Consequently, we have four significant figures.

Again, the number has two digits that are non-zero and are therefore significant figures. Since this is a decimal number with a zero at the end of it, this zero is also a significant figure. 5. 1 0 [-1.2em] Significant Not significant The number has three significant figures.

Round the number to two decimal places.

We are rounding to two decimals. Therefore, we need to look at the third decimal. 0.22↓ 76 Since the third decimal is greater than or equal to 5, we will increase the second decimal by 1. 0.23

Again, since we are rounding to two decimals, we need to look at the third decimal to decide how to round. 2.93↓ 42 Since the third decimal is less than 5, we will keep the second decimal as it is. 2.93

Round to

We want to round to two significant figures. To do that, we start by counting from the left until we reach the second significant figure. 2 5.7409 [-1.2em] First significant figure Second significant figure To round the number to two significant figures, we have to look at the digit that comes after the second digit. 25.↓ 7409 Since 7 is greater than 5, we will increase the second significant figure by 1. Then, we will remove all the numbers to the right of the decimal point. Therefore, we end up with a whole number. 26

Like in Part A, we start by counting the number of significant figures starting from the left. This time, we want to round to four significant figures. 2 5. 7 409 [-1.2em] First significant figure Second significant figure Third significant figure Fourth significant figure To round to four significant digits, we must consider the digit that comes after the fourth digit. 25.74↓ 09 Because the fifth digit is less than 5, we will keep the fourth significant figure as it is and remove the decimals that comes after. 25.74

Find the circle's area and round to an appropriate number of significant figures.

A green circle with a radius of 1.2 meters

The area of a circle is the product of π and the radius squared. A=π r^2 When rounding a calculation, the rule is to round to as many significant figures as we have in the number with the least amount of significant figures. Since π is a number with an infinite number of decimals, we have to look at the value of r. r=1.2 The radius is given with two significant figures. This means we have to round to two significant figures.

The area of the circle is about 4.5 square meters.

Which of the following situations does not require an exact answer?

Let's go through the statements one at a time.

Statement A

When we are measuring distances between two geographical locations, we are usually not interested in the exact distance. Let's think about it — if we drove 19.89 miles, would we say that we drove 19.89 miles or about 20 miles? Therefore, when we estimate how far Kriz drove, it is fine to use rounded numbers. Question: &How many miles did &Kriz travel? Answer: &They traveled about &311+178=489 miles

Statement B

Whenever we pay for something, it has to be exact. We do not want to overpay as a buyer, and the seller does not want us to underpay. Therefore, this requires an exact answer. Question: &How much does Jordan &have to pay? Answer: &She has to pay &4($19.90)-$5=$74.60

Statement C

Here, we are figuring out if a given amount of cash is enough to buy something. To do that, we usually start by rounding the prices to make calculations easier. If we round the prices up and the total is below $12, we know for a fact that we can afford the items. Therefore, the question does not require an exact answer. Question: &Is$12 enough? Answer: &Yes because &$2.20+$4+4($1)=$10.20 &is less than$12.

Levels of Accuracy

Recommended exercises

To get personalized recommended exercises, please login first.
Return to lesson.
Loading content