Sign In
Understanding Similarity Transformations







| | 13 Theory slides |
| | 11 Exercises - Grade E - A |
| | Each lesson is meant to take 1-2 classroom sessions |
Catch-Up and Review
Here is some recommended reading before getting started with this lesson.
- Concept and properties of dilation.
- Concept and properties of rigid motion.
- Concept and properties of congruence.
Investigating Similarity
The applet below shows a spiral tiling of a plane using quadrilaterals of different sizes.
- Moving the left slider dilates the shaded quadrilateral using the center.
- Moving the right slider rotates the shaded quadrilateral around the center.
Move the sliders to match the quadrilaterals in the tiling.

Similarity Transformations
On the previous applet the combination of a rotation and a dilation moved the quadrilateral to match the other quadrilaterals in the tiling. This combination of transformations has its own name.
Similarity Transformation


Properties of a Similarity Transformation
Previously, it was seen that rigid motions keep the figure's size and shape. In comparison, dilations keep the figure's shape but can change its size. The next natural question is, what does a similarity transformation do to a figure?
The following is a list of a few important properties of similarity transformations.
- The image of a line segment is a line segment. The image is longer or shorter than the preimage in the ratio given by the scale factor.
- Similarity transformations preserve angle measures.
Proof
These are properties of both rigid motions and dilations. Since similarity transformations are combinations of rigid motions and dilations, these are also properties of similarity transformations.
Definition of Similar Figures
∼indicates that two figures are similar.


Analyzing Similar Figures
The figure below is put together using 39 similar tiles.

Answer
Example Answer: Translation up, followed by a rotation clockwise by 90 degrees, followed by a dilation using scale factor 2.
Hint
Solution



Once a vertex is at the right place, a rotation can be used to position the pre-image in the right direction.

A dilation by scale factor 2 completes the transformation.

Criteria for Similar Polygons
For polygons, similarity can be checked by considering angle measures and side lengths.
Two polygons are similar if and only if both of the following two properties hold.
- The corresponding angles are congruent.
- The corresponding sides are proportional.
Proof
A biconditional statement can be proven by separately proving the corresponding conditional statement and its converse.
| Conditional Statement | Two polygons are similar if the corresponding angles are congruent and the corresponding sides are proportional. |
|---|---|
| Converse | If the corresponding angles in two polygons are congruent and the corresponding sides are proportional, then the polygons are similar. |
Consider and prove each statement one at a time.
Proving the Conditional Statement
If two polygons are similar, then a similarity transformation that maps one polygon to the other exists. Consider how that relationship affects the corresponding angles and sides of the similar polygons.
- Angles: Similarity transformations preserve angle measures. That means the corresponding angles of the two polygons are congruent. ✓
- Sides: Similarity transformations map line segments to other line segments. The length of all line segments change according to the scale factor. That means the corresponding sides of the two polygons change proportionally. ✓
These observations conclude the proof of the conditional statement.
Proving the Converse
Consider two polygons with congruent corresponding angles and proportional corresponding sides. The proof here will be carried out for quadrilaterals ABCD and PQRS, but it can be generalized to any polygon.


| Observation | Justification |
|---|---|
| P=A′′ | The translation moves A to P and, since this is the center of rotation, it stays there. |
| B′′ is on PQ | This is how the angle of rotation was chosen. |
| D′′ is on PS | This is true, because by assumption ∠A is congruent to ∠P and because rigid motions preserve angle measures. Note that, in this case, the orientation of ABCD and PQRS is the same. If the orientations are different, then a reflection of A′′B′′C′′D′′ in line PQ is also needed to match the orientations of the polygons. |

| Observation | Justification |
|---|---|
| P=A′′′ | The translation moves A to P and, since this is the center of rotation and also the dilation, it stays there. |
| Q=B′′′ | This is how the scale factor of the dilation was chosen. |
| S=D′′′ | Since translations and rotations are rigid motions, AB=A′′B′′ and AD=A′′D′′. It is assumed that PQ/AB=PS/AD, so the dilation that moves B′′ to Q, also moves D′′ to S. |
| C′′′ is on QR | It is assumed that ∠B≅∠Q. Since rigid motions and dilations preserve angles, this means that ∠B′′′≅∠Q. |
| C′′′ is on SR | It is assumed that ∠D≅∠S. Since rigid motions and dilations preserve angles, this means that ∠D′′′≅∠S. |
| R=C′′′ | Both R and C′′′ is the intersection of QR and SR. |
Investigating Similarity of Different Polygons
Determine whether the following statements are true or false.
Hint
Do all quadrilaterals have the same angles?
Solution
Different quadrilaterals may have different angles. Since similar polygons have congruent corresponding angles, this means that not all quadrilaterals are similar.

Hint
Do all trapezoids have the same angles?
Solution
Different trapezoids may have different angles. Since similar polygons have congruent corresponding angles, this means that not all trapezoids are similar.

Hint
Do all parallelograms have the same angles?
Solution
Different parallelograms may have different angles. Since similar polygons have congruent corresponding angles, this means that not all parallelograms are similar.

Hint
Do all rhombi have the same angles?
Solution
Different rhombi may have different angles. Since similar polygons have congruent corresponding angles, this means that not all rhombi are similar.

Hint
Consider the ratio of the sides.
Solution
The corresponding sides of similar polygons are proportional. Since 1:2=3:4, the rectangles on the diagram are not similar.

Not all rectangles are similar.
Hint
Do all squares have the same shape?
Solution
Consider the angles and the sides of a square with side length m and a square with side length n.
- All angles of both squares are right angles, so the corresponding angles of the two squares are congruent.
- The ratio between any sides of the two squares is m:n, so the corresponding sides are proportional.
These two properties guarantee that the squares are similar.

Any two squares are similar.
Solving Problems Using Similarity of Polygons

Hint
Find the length of AD first.
Solution
Focus on the first two triangles to find the length of AD first.

CA=5, BA=4
LHS⋅5=RHS⋅5
ca⋅b=ca⋅b

The other three triangles are also similar to △ABC, so the corresponding sides are proportional.
| Proportion | Solution | |
|---|---|---|
| Expression | Substitution | |
| CAEA=BADA | 5EA=425/4 | EA=425/4⋅5=16125 |
| CAFA=BAEA | 5FA=4125/16 | FA=4125/16⋅5=64625 |
| CAGA=BAFA | 5GA=4625/64 | GA=4625/64⋅5=2563125 |
The length of GA is 2563125, or approximately 12.2 centimeters.
Solving Problems Using Similarity and Congruence of Polygons
In the diagram all quadrilaterals are similar, and the two shaded quadrilaterals are congruent. The length of three sides of the shaded quadrilaterals are 1, w, and w2.

Hint
Write the horizontal base of the top left corner's quadrilateral in two different ways.
Solution
The solution has several steps. First, look at the quadrilateral in the top left corner and compare it to the quadrilateral next to it.




Extra
Exact solutionPractice Using Similarity to Solve Problems
On the diagram all quadrilaterals are similar.

Similarity in the Natural World
There are examples where similar shapes appear in nature.
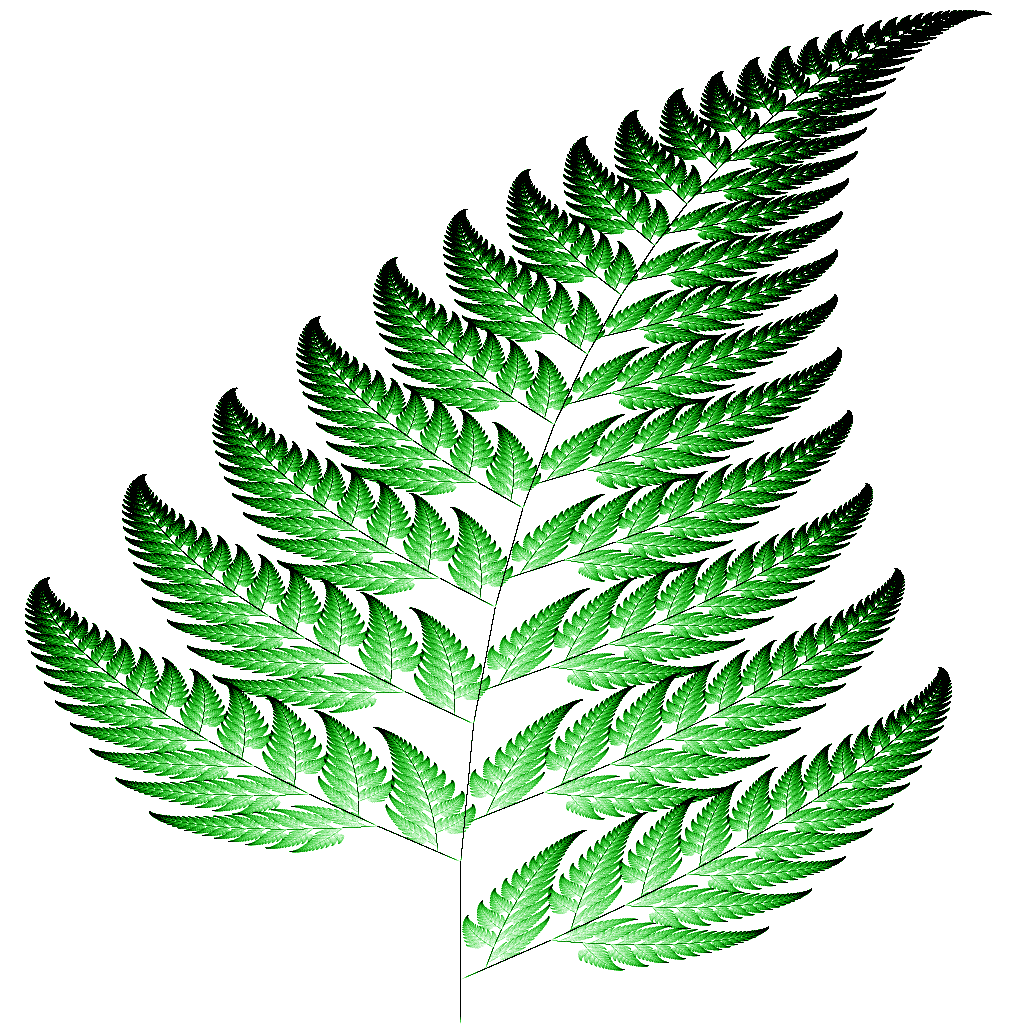
It is interesting to investigate the three-dimensional self-similar nature of a romanesco broccoli. It is built up of parts that are similar to the whole.
Self-similarity is used as an inspiration in fractals. The image below is not a living plant, it is a computer generated image using a construction that uses similarity.
Digital Tools
Python code to draw the imageThe following is the Python source code the author used to draw the image.
import random
import tkinter as tk
width, height = 1024, 1024
pixels = [0] * (width * height) x, y = 0, 1
for n in range(60 * width * height): r = random.random() * 100 xn, yn = x, y if r < 1: x = 0 y = 0.16 * yn elif r < 86: x = 0.85 * xn + 0.04 * yn y = -0.04 * xn + 0.85 * yn + 1.6 elif r < 93: x = 0.20 * xn - 0.26 * yn y = 0.23 * xn + 0.22 * yn + 1.6 else: x = -0.15 * xn + 0.28 * yn y = 0.26 * xn + 0.24 * yn + 0.44 x_pix = int(width * (0.45 + 0.195 * x)) y_pix = int(height * (1 - 0.099 * y )) pixels[x_pix + y_pix * width] += 1 greys = [ max(0, (256 - p) / 256) for p in pixels]
colors = [int(c * 255) for g in greys for c in [g ** 6, g, g ** 6]]
root = tk.Tk()
p6header = bytes("P6\n{} {}\n255\n".format(width, height), "ascii")
img = tk.PhotoImage(data=p6header + bytes(colors))
tk.Label(root, image=img).pack()
img.write("barnsley-fern.png", format='png')
tk.mainloop()Similarity and the Golden Ratio
In the diagram below move the point to adjust the dimensions of the rectangle. Different shapes will be created. Some rectangles are narrow and tall, some are wide and flat. Move the point to create rectangle of any preferred combination.

Well, personal preference is very subjective. Nevertheless, there is a specific ratio that is used often in design. If a rectangle is such that cutting off a square gives a similar rectangle, then the ratio of the sides is called the golden ratio. Such a rectangle is called a golden rectangle.

Composition with Yellow, Blue and Red, a painting of Piet Mondrian, which he painted between 1937 and 1942. Move the slider points to search for golden rectangles in this famous painting!
Consider the similar shapes below.

Corresponding sides in similar shapes are between the same two pairs of corresponding angles. Examining the triangles, we can identify the corresponding sides.
Let's summarize the corresponding sides. AC and DF BC and EF AB and DE
Having identified corresponding sides in Part A, we can determine the ratio of two corresponding sides. Since we should determine the ratio of a side in triangle △ DEF to its corresponding side in △ ABC, the smaller triangle's side should be divided by the corresponding side in the greater triangle.
Remember, because these triangles are similar, when we reduce these fractions, they should be equal. 6/15=8/20 ⇓ 2/5=2/5 The ratio of a side in the smaller triangle to the greater triangle is 25.
In Part B, we found the ratio of a side in the smaller triangle to the corresponding side in the greater triangle. Therefore, the ratio of the hypotenuses must also be 25. To verify, we can determine the lengths of the hypotenuses by using the Pythagorean Theorem.
The greater triangle has a hypotenuse of 25 units. Let's also solve for the smaller triangle's hypotenuse.
The smaller triangle has a hypotenuse with a length of 10 units. When we know both sides, we can find the ratio of the hypotenuses. DE/AB = 10/25 = 2/5
Find the missing length, x, in the similar figures.



We know that the triangles are similar. The shorter side in the smaller triangle has a length of 4 units. In the greater triangle, the shorter side is 6 units long. For the triangles, the corresponding longer sides are 6 and x units long, respectively.
For similar shapes, the ratio between corresponding sides is the same.
x/6=6/4
To find the missing length we will solve this equation for x.
Like in Part A, we first have to identify corresponding sides in the shapes.
We will now solve the equation for x.
As in previous parts, we will identify corresponding sides and write an equation.
As in previous parts, we will solve this equation.
What transformation(s) can show that the figures are similar? Use as few transformations as you can.



Examining the diagram, we see that the triangles have different size. Therefore, we need to dilate one of the shapes to make them congruent.
Size
Let's enlarge the smaller of the triangles until it is congruent with the greater one.
The triangles now have three pairs of congruent angles, and three pairs of congruent sides. Therefore, they are congruent. However, since they have different positions and orientations we need to perform at least one rigid motion to make them map onto each other.
Position and Orientation
By reflecting one of the triangles we can change both position and orientation simultaneously. By having the line of reflection coincide with the side where the triangles meet we can then make them map onto each other after the transformation.
First we notice that we have four pairs of congruent angles, and four pairs of congruent sides. Therefore, these are congruent figures. However, the kites have different positions and orientations which means we need a sequence of rigid motions to make them map onto each other.
Position
Since the figures have different positions, we have to perform a translation in order to make two corresponding vertices coincide.
Orientation
Finally, to make the kites map onto each other, we will rotate one of them about the vertex where they already coincide.
Notice that the circles have different size. This means we must include a dilation in addition to any other transformation we need to make the circles map onto each other.
Position
Let's translate the smaller circle such that the centers coincide.
Orientation
Next, we will rotate the smaller circle such that the white points have the same orientation.
Size
Finally, we can dilate the smaller circle until it has the same size as the greater circle. This makes them map onto each other.
Consider the following similar quadrilaterals.

The two quadrilaterals have different positions, orientations, and sizes. Therefore, to make them map onto each other, we must perform a translation, a rotation, and a dilation on one of the figures. However, it does not have to be in that specific order. To make things clearer we will remove the segment lengths.
Position
Let's translate the smaller quadrilateral until two corresponding vertices coincide.
Orientation
Next, we need to give the quadrilaterals the same orientation. To do that, we must rotate one of them until two of its sides map onto the corresponding two sides in the similar quadrilateral.
Size
Finally, we must dilate one of the triangles to make them map onto each other.
To determine the correct scale factor of dilation, we must divide a side in the greater quadrilateral by the corresponding side in the smaller quadrilateral. Let's identify a pair of corresponding sides.
Having identified a pair of corresponding sides, we can determine the scale factor of dilation k. k=20/10=2
The ratio of the lengths of corresponding sides in similar figures is the same no matter which corresponding sides we compare. With this information, we can set up a proportionality.
Let's solve this equation for x.
Determine whether the statement is always, sometimes, or never true.
Any two regular pentagons are similar.
A hexagon and a triangle are similar.
A square and a rhombus are similar.
Two shapes are similar polygons if two criteria are met.
- Corresponding angles are congruent.
- The lengths of corresponding sides are proportional.
The given statement says that any two regular pentagons are similar. Let's draw two arbitrary regular pentagons.
Since each interior angle of a regular pentagon is 108^(∘), the corresponding angles of any two regular pentagons are congruent. Additionally, a regular pentagon has five congruent sides. That indicates that the corresponding sides' lengths of any two regular pentagons are proportional.
Therefore, it is always true that any two regular pentagons are similar.
As we talked about in Part A, two polygons are similar if corresponding angles are congruent and if the lengths of corresponding sides are proportional. Let's draw an arbitrary triangle and an arbitrary hexagon.
Since a hexagon and a triangle are different shapes, they will never be similar. Therefore, it is never true that a hexagon and a triangle are similar.
Let's recall the definitions of a square and a rhombus.
- Square: A square is a parallelogram with four congruent sides and four right angles.
- Rhombus: A rhombus is a parallelogram with four congruent sides.
The only difference between these definitions is that for squares, all angles have to be right angles. Therefore, we know that the relationship between a square and rhombus' corresponding side lengths are proportional. Additionally, when a rhombus has four right angles, its corresponding angles are congruent.
Therefore, it is sometimes true that a square and a rhombus are similar.
Are the following polygons similar?



Shapes are similar when two criteria are fulfilled:
- Corresponding angles must be congruent.
- The lengths of corresponding sides are proportional.
Examining the diagram, we see two triangles, each with three congruent sides. This means these are equilateral triangles. Such triangles also have three congruent angles, each with a measure of 60^(∘). Let's add this to the diagram as well as the ratio of corresponding sides.
Since the triangles have three pairs of congruent angles and the ratio of the triangles' corresponding sides are proportional, the triangles are in fact similar.
Examining the diagram, we notice that the quadrilaterals have four pairs of congruent angles. However, this is just one criterion. We must also make sure that the ratio of corresponding sides are equal for all corresponding sides.
Let's reduce the fractions as far as we can to check if they are equal. 3.6/7.2? =3/6? =5/7 ? = 6/9 [0.5em] ⇓ [0.2em] 1/2=1/2≠5/7≠ 2/3 * As we can see, the ratios of what would be corresponding sides are not equal. Therefore, these are not similar quadrilaterals.
To determine if two triangles are similar we need to know at least one of the following information:
- At least two angles in both triangles.
- All of the sides in both triangles.
We have only been given a right angle in these triangles. However, we do know all of the sides in both triangles. With this information, we can determine the ratio of corresponding sides. If these are similar triangles, the smaller legs are corresponding, as are the longer legs and hypotenuses.
Let's calculate the fractions to check if they are equal. 33/8 ? =56/15? = 65/17 [0.5em] ⇓ [0.2em] 4.125≠ 3.733... ≠ 3.823... * We can conclude that the triangles are not similar.